Correcting a Newtonian

Part of the reason that contrast is so bad on my Newtonian is that it has flare. The other reason is that it is uncorrected. Let's see what it takes to correct the thing.

The mirror is a 300 mm diameter diffraction-limited 1/4 wave parabolic mirror. Sounds awesome, especially the diffraction limited part. When viewing 550 nm (green) light, a 300 mm aperture scope should resolve 1.22*wavelength/diameter = 2.2 microradians. With a 1500 mm focal length, those details are 3.4 microns across on the image plane. My Canon 40D has 5.7 micron pixels, which isn't quite going to catch the details.

Sadly, it turns out that even a perfect parabola produces just a single perfectly focussed dot in the center of the image, and resolution goes downhill out from there. One way to measure resolution is to measure the amount of contrast transmitted by the lens at a particular spatial frequency. We could measure transmission at the diffraction-limited spatial frequency (227 line pairs/mm), but we don't need it to be that good. A more useful frequency is the maximum spatial frequency that the camera supports. The pixels themselves sample 87.7 lp/mm. Because the camera has a Bayer filter to sample colors, it has an antialiasing filter, and the maximum frequency it can sample correctly is a factor of 1.8 smaller, about 48.7 lp/mm.

Here's a graph of the contrast you'd expect to transfer, at both 227 lp/mm (diffraction limit) and 48.7 lp/mm (Canon 40D limit). You can see that resolution from a simple parabolic mirror is only good within a small image circle around the center, and there is almost no contrast available at the diffraction limit.

Once contrast drops to zero, there is no detail left at that frequency. Lower spatial frequencies, corresponding to less detailed imagery, will have contrast at larger and larger radii, and so the picture will look more blurry as you get farther from the center. For photographic lenses, I like to see MTF at the maximum camera frequency of something like 30-40% across the whole field, although I'm willing to accept some dropoff at the corners.

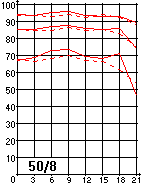Compare the parabolic mirror graph to a similar graph for the Canon 50mm/1.4 lens, in particular, looking at the 40 lp/mm line (the bottom pair). 60-70% MTF. That's a good lens. (I got this graph from photodo.com, which has great data on hundreds of lenses.)

The graphs aren't perfectly comparable, but they're close. The Canon here is stopped down to f/8, which vignettes away the least corrected portion of the aperture. But it performs nearly as well at f/5. Also, this graph is at 40 lp/mm, which is a little easier than the 48.7 lp/mm I'm using to judge the Newtonian. One other detail: in both graphs, there are two lines, one dashed (tangential) and one solid (saggital). Saggital means "in the direction towards and away from the image center", and tangential means"along a curve centered at the image center".

So the reflector looks terrible, but there is hope. Al Nagler at Tele Vue has designed a corrector lens (the Paracorr) that, when combined with a parabolic mirror, gives a well corrected image. I don't have the Paracorr's prescription (I checked the U.S. Patent Office, and found Al's eyepiece patents but no patent for the Paracorr), but I know the basic idea, so I was able to slap something together with Zemax to demonstrate.

Here is the overall scheme: light comes in from infinity from the left, bounces off a parabolic mirror at the far right, and then comes back through a negative doublet, followed by a positive doublet, finally arriving at the image sensor at the far left. I've left out the planar secondary miror that reflects the light out the side, but I've left in the obstruction that it causes. The corrector assembly, as shown, sits just outside the main optical tube. The eyepiece would sit on the other side of the image plane.

When comparing this to a photographic lens, it's best to think of the mirror combined with the two doublets as being the "lens". It actually extends the focal length of the telescope a bit. This picture shows rays bouncing off the primary mirror, going through the two doublets, and arriving at the image plane. Because the doublets are so small relative to the mirror and focal length, it's hard to see the detail.

So, here's the detail. I'm quite pleased with how this turned out: the elements are not ridiculously thick, and there is 50 mm of clearance between the last element and the focus plane, good enough to mount a DSLR (44 mm clearance required) if not a T thread mount (55 mm required). The negative doublet is a bit too close to the mirror, in the sense that it would probably sit right at the edge of the main optical tube, when we'd prefer it to be back a bit so that we can baffle the focus tube so that stray light from the front opening of the tube can't speckle off the doublet.

Designing this wasn't too hard. I left Zemax running overnight doing a global search for an optimum, with no constraints on the glass choice. A real optical designer has more constraints to deal with.

And here's the resulting MTF, polychromatic, no less! This is pretty incredible, I doubt the real Paracorr is this good. It's slightly better, all the way across the field, than the Canon 50mm/1.4. That's astonishing, given that this thing has 7 surfaces (one aspheric -- the mirror) with which to bend the light, compared to 13 in the Canon refractor. Or, compare this to the plot at the top of the post for the performance of the parabolic mirror alone. Night and day.

I think the bottom line is that a Paracorr is a necessary part of a Newtonian telescope, unless it's only used at very high magnifications. Unsurprisingly, the Paracorr is the best-selling product made by Al Nagler's company.

Light bucket

I have a a 12 inch Newtonian telescope on a Dobsonian mount. It's a cheap light bucket. Over the weekend I tried hooking up a DSLR camera to it.

Two initial results:

1. The adaptor physically connects the DSLR to the scope, but it doesn't guarantee that it will work. In my case, it doesn't. The problem probably applies to most telescopes designed to be used with eyepieces:
• The eyepiece, e.g. a 30mm eyepiece, is a 30mm focal length lens designed to take an image 30mm in front of the lens and make it appear to be at infinity. Your eye looks through the lens to see the image at infinity.
• The image that the eyepiece is focussed on is 30mm in front of the first nodal point of that lens. It appears that the standard for telescope eyepieces is to have that image in front of the shoulder of the eyepiece. When you remove the eyepiece and put a plain piece of paper on the image, you find that it is 5-10mm inside the focussing tube.
• The focussing tube has some range, such that your can rack it forward and get the image to be 10mm behind the focussing tube, but:
• DSLRs all want about 42mm between their front flange and the sensor plane. You focus them by placing the image on the sensor plane.
• There is no way to focus a camera attached to this thing at infinity, without altering the telescope to move the image focus out.
• If the image focus is moved out, some sort of extension tube, about 2 inches long, will be necessary with all eyepieces to make it possible to focus them.
• I'll simulate it, but I suspect that extension tube will then limit the field of view of some of the larger FOV eyepieces.
2. Surprise, the DSLR will focus on things at finite distances! If you pull the imager on a 1500mm scope out 2 inches from focussed-at-infinity, you are focussed 43.5m away. So, I took some shots of some tree branches at about that distance while pointed close to the sun.
• This was a little dangerous, because if I'd accidentally pointed it at the sun while looking into it I could have hurt myself. I got lucky this time, and I'll not be impatient again.
  
• Depth of field is awful. Spot size is about 10 microns (pixel size x 1.8 for the Bayer sensor), so an f/5 scope focussed at 43.5m away has a depth of focus of +/- 5*10 microns which corresponds to +/- 45 mm out by the tree branches.
  
• The focus was only okay, not great.
• Global contrast issues below
• The scope has, at minimum, nasty coma which will smear images. I had a Paracorr lens between the camera and the telescope, but I don't think I had it adjusted properly, and I have not verified that the scope actually has a parabolic and not spherical mirror.
  
• Contrast was ridiculously bad. If you saw a photographic lens this bad you might chuckle, but you would never, ever consider buying it. Saturday night I tried looking at the moon, and found that anywhere within 20 degrees of the moon the sky had a uniform grey background that hid most of the stars.
• I need to clean my optics, there is dust on them.
• I need to flock the interior of the telescope.

Maybe amateur telescopes all have terrible contrast because amateur astronomers are used to looking at stuff that's mostly black, so that a little scattered light is no problem, compared to daytimes scenes where it does matter.

So, photography through the telescope is not a trivially implemented idea. It does have me thinking about how to design a Newtonian telescope that can do photography, daytime or night, as well as stargazing. I know a thing or two about flare suppression and camera design as a result of my work on Street View, and I can see a bunch of obvious problems that might be fixable.

• The secondary mirror is not balanced on the spider, so that it twists as the telescope is changed in altitude. I can actually see this with the autocollimator in the scope, which measures maybe 4mm of drift between horizontal and vertical. That's an angle of 2.67 milliradians. The autocollimator doubles the actual angle, so it's about 1.33 milliradians. Across the 43mm field of a DSLR, that's 57 microns of tilt, which is a smidge more than half the focus budget of +/- 50 microns. It would be good to balance the secondary on the spider with a counterweight.
  
• The eyepiece should not view the opposite side of the tube around the secondary mirror. Instead, it should view a recessed surface which is itself shaded from both the aperture and the mirror.
• The eyepiece tube should have a baffle, which is recessed from the tube so that it is not lit by the aperture, and which prevents the eyepiece from seeing anything but the secondary mirror and the recessed light trap behind it.

Hmm.... this is looking like a lot of work.

Honda S2000 vs BMW 330i ZHP

Yes, I know, apples vs oranges.

Martha has not liked my S2000 since I got it, at first because it's loud and has a hard ride, and more recently because it has no back seat. She wanted me to switch to something with 4 doors. We toyed with the idea of getting a Prius for a while, until I realized that I wasn't going to be happy with anything that couldn't wag its tail on dry pavement.

It may seem odd to be comparing these two (or three) cars. I'm not trying to figure out which is the best car in a particular category, rather, I'm trying to figure out which category I want. I decided that I could live without the convertible top, but not without that sense of engagement I get while driving.

The BMW has most of that engagement, so I got that. It's a used 2004 model year car. I am much happier buying a used car than a new one. I bought the Honda (a 2002) new because at the time the new ones were only a couple thousand dollars more than the used ones. The resale value has held up well enough that it should end up costing about $13/day (depreciation, gas, insurance, tires, and maintenance), which is about what I think I should be paying for a car. Because the BMW is used, it will hopefully depreciate at about the same dollar rate even though the car was more expensive new. The Prius would have been a lot less expensive.

Both cars claim almost exactly the same peak horsepower, though the BMW is 500 pounds porkier. Even so, it accelerates faster, because I'm not willing to thrash the Honda's clutch, and because the broader torque curve makes it much easier to be in the right gear in the BMW. The BMW engine feels more practical; it can relax, and it can lunge, and it is inline-6 smooooth. The Honda engine is more exciting, and responds quicker. Where the BWM takes maybe 200 ms between throttle lift-off and actual engine braking, the Honda's delay is unnoticeable -- maybe 50 ms. Throttle-on delay is tiny in both engines. The Honda sounds better, too, and between 6000 and 9000 RPM it is literally in a class by itself. I wish Honda had built a 9000 rpm inline-6 for their car(s). Fuel efficiency scales with body mass, as usual: almost all cars eat their weight in gasoline every year. Just think about that the next time you are considering buying a big pickup.

The Honda transmission is better. The throws are easier and much shorter, it's less rubbery, and it feels better going into gear. I am really going to miss this shifter. The BMW has wider spaced gears (the ZHP comes with a 6-speed manual), which would be a disaster on the Honda because of the peaky torque curve but in this car give a very relaxed engine note on the freeway. A mechanical engineering friend told me that the Honda transmission is probably the best ever made for a production car, so everything else is a step down. That's the problem with really nice stuff -- transitioning away.

The Honda steering is better. The ratio is faster, and it's lighter. The BMW tends to kick back approaching stop signs with uneven pavement. This last issue is probably due to the heavier car on wider rubber on the BMW. The BMW steering wheel is thicker and nicer to hold.

I haven't really pushed the BMW around yet, and I never did push the Honda past its cornering limits, but I can say that the very first thing I liked about the Honda is still true: there is less commitment in corners. When you are tearing around a bend, you can change your mind, change your line, get into the brakes, roll on the gas, you can do all kinds of things and the Honda reacts in a predictable manner. The BMW feels committed, and that feels scary. Operators vill not exceed zeez limits.

The BMW has a nicer interior than the Honda. It's quieter, the stereo is better, the instruments tell you your averaged MPG, and there is more room. The pedal position is way better than the Honda -- you can really toe-and-toe in the BMW; the Honda pedals were too far apart to do that reliably. Toe-and-toe'ing is lame, though, compared to heel-and-toeing. The best pedals I ever had were in my VW Bug. Yes, the car had a lot of other problems (it rusted all the way through the roof in one spot, the gas pedal would occasionally stick to the floor, and the steering oscillated badly at 70 MPH) but I've never driven any other car in which I could reliably match revs while under hard braking.

I like driving the new car, and I've taken all three girls in it now and they like it. (Well, Ava is noncommital, but she is just one year old and hasn't yet developed her appreciation of these things.) Anya yells "two wheels" going around brisk corners in this one too, although I sometimes think I hear a wistful note in her voice. Ah well, life goes on. I'm sure the next car will be a barge.

Lady Jane

We have a new puppy, Lady Jane.

She's a black Lab, just like Iniki was. She's really cute and full of sharp teeth.

Chernobyl, ghost town

Elena here is holding a geiger counter reading 763 microroentgen/hour. In the background is the sarcophagus built around the reactor that exploded in Chernobyl. Normal radiation levels are 10-20 uR/hr. She has an interesting photo tour of Chernobyl here.

The sarcophagus looks a lot better than I expected it would.